Numerical Investigation of Droplets Moving on Inclined Surfaces: Forces Acting During Motion
Bodziony, Francisco_Numerical Investigation of Droplets Moving on Inclined Surfaces: Forces Acting During MotionFigure 1: Viscous force density field, viewed from the droplet’s contact area.
Francisco BodzionyIntroduction
Despite the motion of drops seeming a simple phenomenon, the behavior of a droplet on an inclined solid surface is far to be completely understood. The quantitative and qualitative description of the forces acting on droplets during their motion is fundamental in order to understand how the droplet interacts with the surface. Despite this, there seems to be a lack of knowledge in quantifying the forces in a sliding droplet, mainly near the contact line. Thus, we simulate via direct numerical simulations (DNS) the motion of water and water-glycerol (85% wt.) droplets on inclined surfaces. The objective is to gain insight into the capillary and viscous forces that act on the droplet, mainly their influence and their contribution to the overall motion. These results are then compared to experimental observations and existing correlations.
This project is funded by the Deutsche Forschungsgemeinschaft (DFG, German Research Foundation) - Project-ID 265191195 - SFB 1194.
Methods
The open-source C++ library OpenFOAM® is used. We have implemented a phase-field multiphase flow solver, where the coupled Cahn-Hilliard Navier-Stokes equations are solved. The multiphase solver, named phaseFieldFoam, has been developed for OpenFOAM (FOAMextend 4.x/5.0). Phase-field methods model the interface, which separates two fluid phases, as a continuum transition with small width by continuously changing the phase-field order parameter (or volume fraction) from one phase to the other. Here, the interface motion is driven by the gradient in the local chemical potential - such an approach inherently regularizes the stress singularity at the moving contact line.
Results
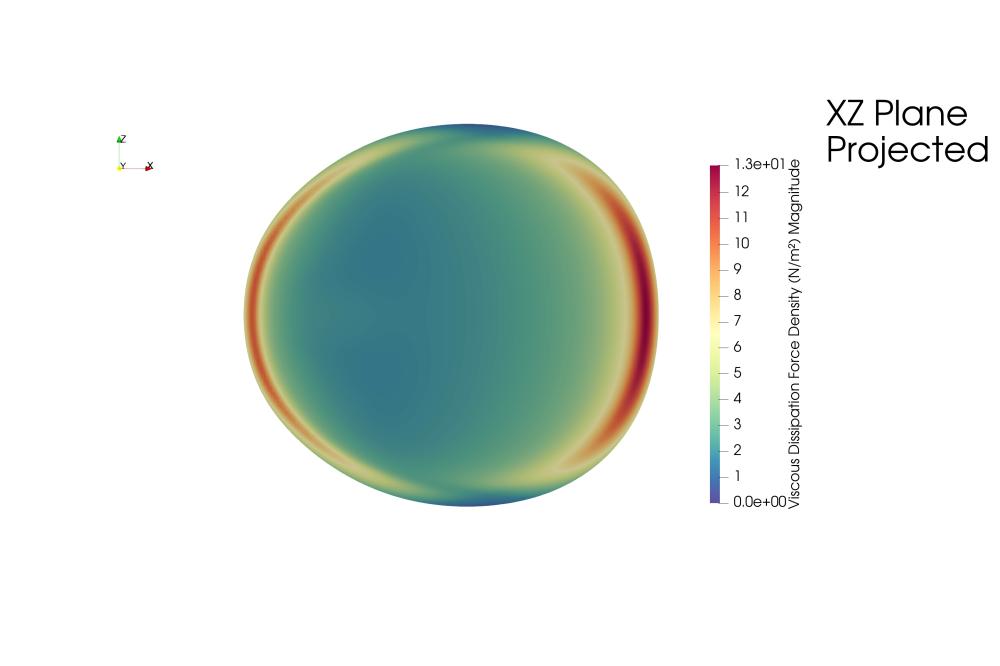
Validation is achieved by quantitatively comparing the experimentally obtained steady-state velocity of water-glycerol (85% wt.) droplets at various substrate inclinations with the ones from coming from DNS, where deviations lower than 10% are obtained. For water droplets, a discrepancy between the simulated and estimated viscous dissipation forces is observed, which may be attributed to the misused fitting parameter used for estimating such forces. Moreover, the viscous dissipation force field is found to be non-uniform along the droplet’s contact line perimeter (Figure 1). For glycerol droplets, there is a satisfactory agreement between correlated and simulated forces, leading us to the conclusion that special care must be taken when employing such correlations, especially for faster moving droplets. Here, we observe that the wedge viscous force is the largest contribution to the total force counteracting the droplet motion.
Discussion
In this work the contribution of forces during the droplet motion with varying liquid viscosity and contact angle has been investigated numerically. This study not only provides insights into the flow inside moving droplets with relevance to e.g. microfluidic processes, but also can help the design of such processes.
Outlook
In technical applications and nature, surfaces do present some sort of heterogeinity (whether chemical or geometrical). As such, our next focus is to continue to investigate the forces that act on moving droplets which move on complex substrates.
