Reliability of Statically Indeterminate Concrete Beams With GFRP Reinforcement
Introduction
Structural engineering, particularly concrete systems, has long focused on understanding the distribution of internal forces within continuous structures. However, while considerable research has been conducted on the behavior of steel-reinforced concrete elements, there is a notable gap in the study of statically indeterminate systems reinforced with fiber-reinforced polymer (FRP). The inherent linear-elastic properties of FRP cause an unanticipated increase in internal forces within the highly reinforced sections of continuous structures. This research attempts to investigate whether the design of such elements based on linear-elastic force distributions leads to safety deficiencies.
This gap in understanding the behavior of FRP-reinforced statically indeterminate systems raises critical questions about their structural integrity. By investigating the effects of linear elasticity on the internal force distribution, this study seeks to uncover potential safety deficiencies and provide insight into optimizing design methodologies for improved structural robustness.
Methods
The methodology employed in this research centers on the development and validation of a sophisticated finite element model specifically tailored to the simulation of FRP-reinforced continuous systems. Rigorous verification against experimental investigations ensures the accuracy of this model. Extensive parameter studies are carried out with the aid of the FE model in order to investigate the various factors influencing the redistribution of internal forces in statically indeterminate systems with FRP reinforcement.
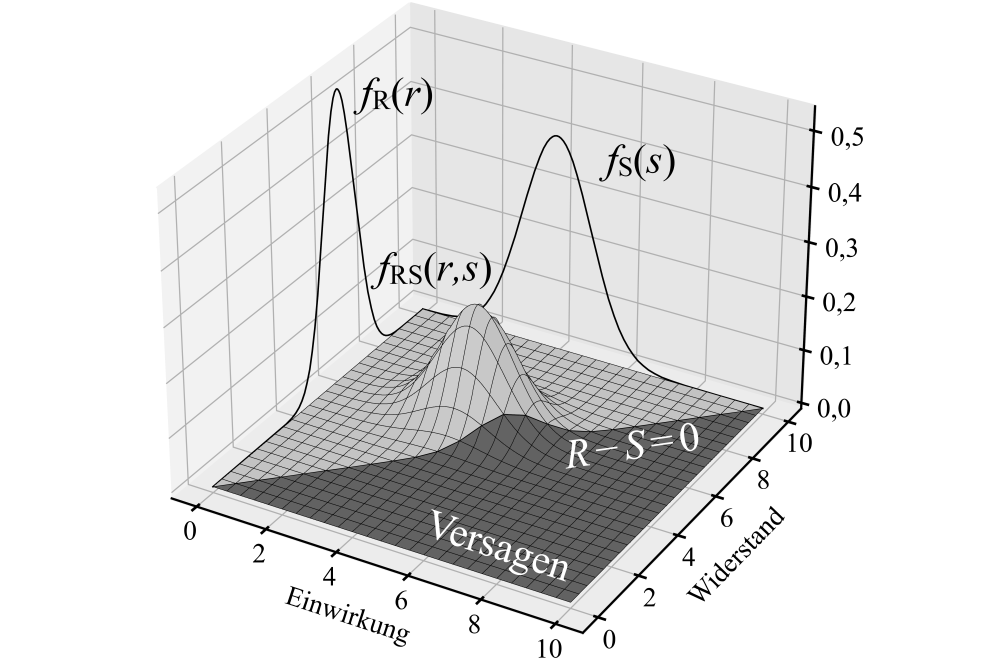
In order to comprehensively analyze the structural reliability, Monte Carlo simulations are used, employing advanced sampling techniques such as Latin Hypercube Sampling and Adaptive Importance Sampling. Extensive reliability analyses are carried out to investigate in detail the reliability of statically indeterminate systems with FRP reinforcement under different boundary conditions.
Results
The investigation provided compelling evidence that the inadvertent redistribution of internal forces in FRP-reinforced systems results in a reduced margin of safety compared to statically determinate structures. However, it was observed that when structural failure is attributed to reinforcement failure, the partial safety factor of the FRP reinforcement can offset these concerns. Conversely, in cases of concrete failure within the member, this compensation mechanism proves to be inadequate. Consequently, it is suggested that concrete failure should be excluded from the design considerations of such components.
These findings not only underscore the complexity of FRP-reinforced systems, but also emphasize the critical need to reevaluate design approaches and safety considerations for such structures.
Discussion
The Monte Carlo simulations performed in this study have yielded promising results that shed light on the intricate interplay between linear elastic material behavior and structural safety in FRP-reinforced systems. However, these simulations open the door to further exploration, such as investigating alternative failure modes, e.g. shear failure, exploring different section geometries such as T-beams, and investigating the reliability of concrete members strengthened with textile reinforced concrete.
Diversifying the scope of analysis beyond the current parameters can provide deeper insights into the structural behavior of FRP-reinforced systems, paving the way for more comprehensive design guidelines and strategies to enhance structural resilience.