Micromagnetic Simulation on SmCo5-Cu Nanocomposites to Investigate Influence of Amorphous Phase
Eren Foya_Micromagnetic Simulation on SmCo5-Cu Nanocomposites to Investigate Influence of Amorphous Phase_Figure1Figure 1: Simulated initial hysteresis curves for (a) varying nanocrystal size (lnc) with constant volume fraction (φnc) = 0.3; (b) varying volume fraction (φnc) with constant (lnc) = 20 nm; (c) micromagnetic models for specific case volume fraction (φnc) of 0.3 and nanocrystal size (lnc) of 20 nm at specific magnetic fields (Hext) at (c1) 0.0 T, (c2) 0.8 T, (c3) 5.0 T, (c4) 6.5 T and (c5) 8.0 T(Ms is valued as the maximum magnetization of each hysteresis).
Reprinted from Scripta Materialia, Vol. 240, Staab, F.; Yang, Y.; Foya, E.; Bruder, E.; Zingsem, B.; Adabifiroozjaei, E.; Nasiou, D.; Skokov, K.; Koch, D.; Farle, M.; Dunin-Borkowski, R. E.; Molina-Luna, L.; Gutfleisch, O.; Xu, B.-X.; Durst, K., Influence of amorphous phase on coercivity in SmCo5-Cu nanocomposites, Cpoyright (2024), with permission from Elsevier.
Yangyiwei Yang
Eren Foya_Micromagnetic Simulation on SmCo5-Cu Nanocomposites to Investigate Influence of Amorphous Phase_Figure2Figure 2: Simulated second and third quadrant of the hysteresis curves for (a) varying nanocrystal size (lnc) with constant volume fraction (φnc) of 0.3; (b) varying volume fraction (φnc) with constant nanocrystal size (lnc) of 20 nm; (c) micromagnetic models for specific case volume fraction (φnc) of 0.3 and nanocrystal size (lnc) of 20 nm at specific magnetic fields (Hext) at (c1) 0.0 T, (c2) -0.9 T, (c3) -4.6 T, (c4) -5.5 T and (c5) -6.9 T (Ms is valued as the maximum magnetization of each hysteresis).
Reprinted from Scripta Materialia, Vol. 240, Staab, F.; Yang, Y.; Foya, E.; Bruder, E.; Zingsem, B.; Adabifiroozjaei, E.; Nasiou, D.; Skokov, K.; Koch, D.; Farle, M.; Dunin-Borkowski, R. E.; Molina-Luna, L.; Gutfleisch, O.; Xu, B.-X.; Durst, K., Influence of amorphous phase on coercivity in SmCo5-Cu nanocomposites, Cpoyright (2024), with permission from Elsevier.
Yangyiwei YangIntroduction
Along with technological advancements, the search for better-performing functional materials is also deepening. Having one of the key roles among many applications, demand for high-performance permanent magnets is rising. SmCo-based magnets are good candidates for permanent magnets since they can withstand complex chemical environments and show stable properties at elevated temperatures. To fulfill the requirements for these magnets, good magnetic properties need to be fulfilled and for that, the micro- or nanostructure needs to be adjusted accordingly. In this work, micromagnetic simulations based on the experimentally observed microstructure are conducted; to clarify the effect of amorphization in a SmCo5 phase and reveal the drawbacks of the amorphous phase. Micromagnetic simulations show the mechanisms of magnetic saturation and the decline of magnetic hardening due to the strain induced by high-pressure torsion.
Methods
Micromagnetic simulations based on experimental microstructural characterizations are conducted to understand the behaviour of magnetic properties in a sample undergoing rotation by high-pressure torsion. Micromagnetic simulations are based on the free energy equation which includes various magnetic interactions, parameters and magnetic properties. Simulation domains are created that contain an elliptical nanocomposite region and a Cu-coated region surrounding it. The domain is equivalent to an intersection of a long elliptic cylindrical structure with columnar nanocrystals. SmCo5 nanocrystals were generated inside the nanocomposite region with controlled nanocrystalline size and volume fraction of nanocrystals., where the inplane domain configuration and domain wall migration are addressed. Effects of size and fraction on the magnetic properties were then separately examined. With the help of micromagnetic simulations, the effect of the amorphous phase and the embedded nanocrystals can be understood on a length scale, at which an experimental investigation is very difficult.
Results
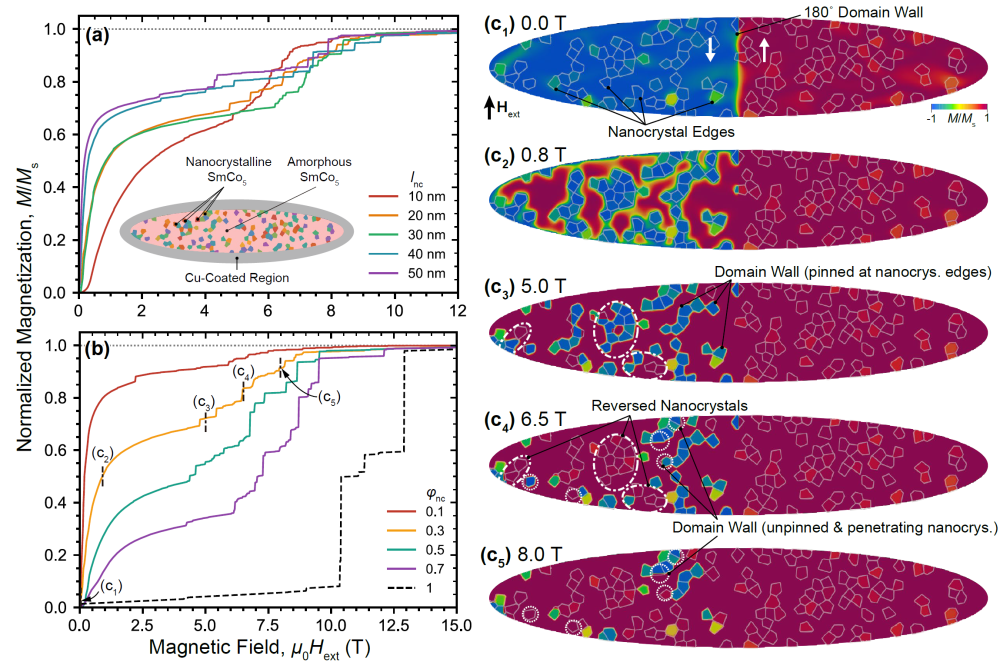
Initially, the micromagnetic simulation of the magnetization processes at different external fields is plotted for one specific case of volume fraction of nanocrystals and nanocrystal size to be able to understand more precisely, how initial magnetization occurs in a microstructure of amorphous matrix and embedded nanocrystals. The specific case, the size of the nanocrystals being 20 nm and the nanocrystalline volume percentage being 30% are from microstructural TEM data. Figures 1 and 2 show simulated hysteresis curves initial magnetization and demagnetization, respectively. To investigate the effects of size and fraction on the magnetic properties separately, on one side, the average nanocrystal size is fixed at 20 nm while the volume fraction of nanocrystals varies as 0.1, 0.3, 0.5, 0.7 and 1(Figures 1a and 2a). On the other side volume fraction of nanocrystals is fixed at 0.3 while the average nanocrystal size is varying as 10, 20, 30, 40 and 50 nm (Figures 1b and 2b ). Both Figure 1c and 2c also show the micromagnetic modelling for specific case φnc = 0.3 and lnc = 2nm at various externally applied magnetic fields (c1, c2, c3, c4 and c5 on both figures).
Discussion
The specific case-having 0.3 as nanocrystal volume fraction (φnc) and 20 nm as average nanocrystal size (lnc)- shows, that with an external field applied the domain wall is pinned at the nanocrystal boundaries, which causes the jumps in the magnetization hysteresis curve. By increasing the strength of the field, the domain wall gets unpinned at the boundaries of the nanocrystals and can penetrate. If the magnetization of the nanocrystal points in the direction of the external field, the domain grows within a group of neighbouring crystallites. The amorphous phase is disadvantageous for improving the magnetic properties due to its significantly worse intrinsic magnetic property (because of the lack of crystallinity) than nanocrystals’. Decreasing the size of the nanocrystals leads to an increasing coercivity for the fixed volume fraction of nanocrystals, since the number of crystal boundaries increases and hence pinning sites with decreasing size of nanocrystals. However, the magnetization reversal of the nanocrystals starts at negative fields that are larger than the coercivity for all cases, meaning that the pinning effects on the nanocrystals boundaries have no influence on the coercivity. For a constant size of the nanocrystals, an increasing volume fraction of the nanocrystals leads to an increased coercivity. The highest coercivity is reached if the particle does not contain an amorphous phase, meaning that the amorphous phase is disadvantageous for improving the coercivity due to its significantly lower magnetocrystalline anisotropy (because of the lack of crystallinity) than nanocrystals’. For small-volume fractions of nanocrystals, the coercivity mechanism is nucleation-controlled and changes to pinning for a complete nanocrystal structure. With increasing volume fraction of the crystallites, it is also obvious that higher fields are necessary to reach magnetization saturation. It is observed that the influence of the volume fraction of nanocrystals is much more distinct compared to the influence of the nanocrystallite size. The reason is the fact that the pinning effects of the nanocrystal boundaries for the small volume fractions of nanocrystals only start at external field strengths which are higher than the coercivity.
The simulated coercivities fit well with the experimental results. In particular, the size of the nanocrystals between lnc = 10 nm and lnc = 20 nm and the nanocrystalline volume percentage of φnc = 0.3 are in good accord with the microstructural data made by TEM. The effect of the amorphous phase and the embedded nanocrystals may be studied on a length scale at which an experiment is highly challenging with the help of the micromagnetic simulations. The simulations show that domain wall pinning occurs at the nanocrystal boundaries and that the amorphous phase is unfavorable for achieving a high coercivity. Since pinning happens at negative external fields that are greater than the coercivity, it has no influence on the coercivity at nanocrystal borders. The simulation results, which highlight the detrimental impact of the amorphous phase, indicate that modifications to the processing are required to reduce the size of the SmCo5 particle to the single domain region and inhibit the amorphous phase at the same time.
