We made several numerical calculations regarding the model of Spatial Random Permutations (SRP). This is a model in statistical mechanics and is of recent interest. In this model we look at  random permutations of lattice points, which can also be identified as a directed Graph, where  each point is connected with its target point via an arrow, such that cycles in the permutation form loops in the graph. As typical in models in statistical mechanics the probability of a certain realization depends on the energy of the system, where the energy and probability of a  realization are defined in such a way that short arrows are more likely. The degree to which  long arrows get penalized depends on the parameter alpha of the system.

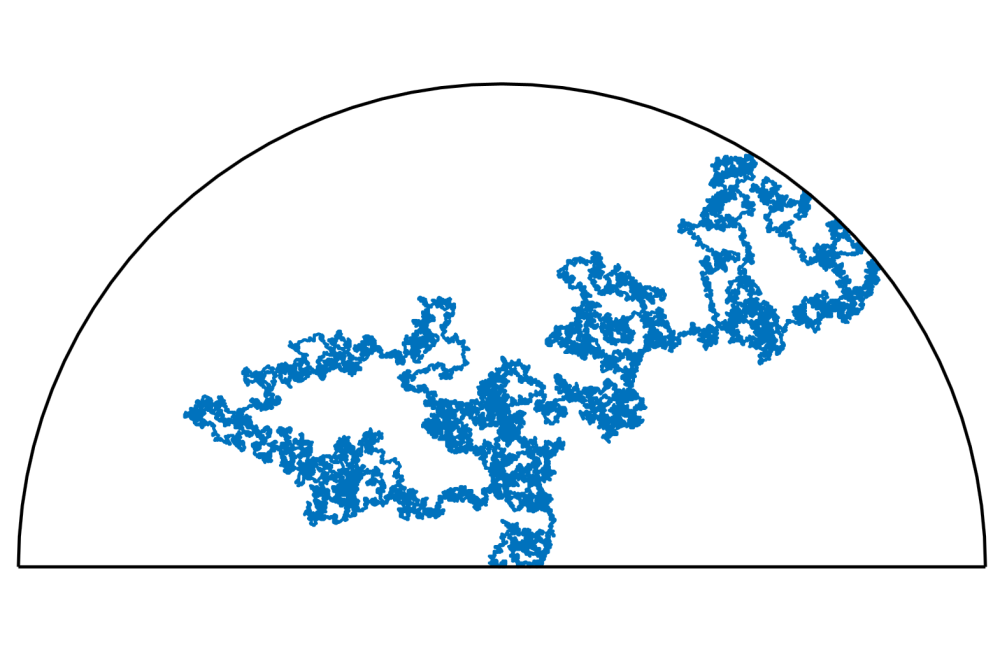
We modify this model in the way that we force a path in the system which starts at the origin  and goes to a boundary point. We believe that there is a connection between this model and  the concept of Schramm-Loewner Evolution (SLE), which is a certain family of random curves in  the complex half plane. The SRP model is hard to handle analytically, so we want to show some connections via numerical calculations. Because we want to look at geometrical quantities and therefore have to look at large systems to avoid finite effects, we need a lot of computation power. The main quantity we look at is the probability for a path in the SRP model to pass left to a certain point in a reasonable sense. In the case of SLE this probability is given by Cardy's formula.